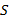Servicios personalizados
Español (pdf)
Articulo en XML
Referencias del artículo
Enviar articulo por email
Citado por SciELO 
Similares en
SciELO 
Permalink
Introduction
Cuban rum is derived from the fermentation and distillation of sugar molasses (a by-product of sugar cane industry). Distillation columns in continuous process are used to produce the distilled spirit under specific organoleptic parameters which guarantee the optimal quality of the final product. The distilled spirit (as primary/raw rum) known in Cuba as “Aguardiente”, is a colorless liquid with typical organoleptic features such as strong characteristic aroma and intense flavor. Therefore, the aguardiente is aged in barrels of white oak wood (Quercus Alba) during a timed period in order to transform and improve its sensorial characteristics.1,2
By the ageing process, the aguardiente changes its original tough sensorial attributes: a light amber color appears; taste softens and a pleasant aroma is produced. During the ageing stage; these sensorial changes are obtained by complex chemical reactions derived from the interaction between the aguardiente and the oak wood.3 Constituents in the wood are transferred to the bulk liquid in the barrel by mechanisms such as diffusion, convection currents in the bulk liquid, and temperature cycling. As the barrel heats up, the gas above the liquid increases in pressure and forces liquid into the barrel wood. When the barrel cools and the gas pressure drops, the liquid flows out of the wood back into the bulk liquid, thus carrying the wood constituents.1,2 Additionally, the concentration of flavors and aromas in aged rums is also a consequence of evaporation which in turn is an important factor in maturation.4
In that sense, the volume loss is an inherent part of the ageing process. Due to its porous structure, the barrel acts as a semi permeable membrane that allows evaporation from the cask and migration of air into the barrel.1 Environmental conditions in barrel storage facilities are frequently not controlled, which leads to differences in ageing effects in the variety of climatic conditions in which spirits are aged. The annual percent of volume loss mainly depends on factors such as climatic conditions of the cellar (air humidity, velocity, and temperature), wood and liquid properties (porosity, density, stave preparation, barrel size and morphology of the wood fiber) which have a significant influence.5,6 The spirit evaporation during the ageing process is an unquestionable economic issue for producers. Therefore, efforts to diminish and/or control the loss during ageing are crucial in order to optimize the production process and to reduce the associated costs. The most frequent solutions for diminishing the evaporation loss are focused on temperature and humidity-controlled cellars. 1,5 Apart of the previously mentioned factors, there are other aspects to take into account in order to reduce the volume loss during rum ageing. In order to increase the storing/logistic efficiency of the cellar’s space, spirit producers have introduced the palletized system of ageing which consist on storing the barrels in vertical position and supported by pallets (figure 1).
Fig.1 Palletized ageing system. Barrels are stored in vertical position. (picture with the permission of the major rum producer of Cuba)
Palletized strategy has advantages compared with the traditional racked ageing system (barrels horizontally stored). The vertical arrangement not only increases the volume storage yield (L/m2) in cellars up to 20% but also improves the work system dynamics in the cellar, which in turn impact significantly on the logistic/production capacity of the spirit manufacturing. However, after many years of using the traditional rack-system of ageing, some aspects still under assessment during rum ageing in palletized system. A general structural diagram of a wood barrel is presented in figure 2(a). Traditionally, as the barrel is prepared for rack ageing system, a bung-hole is placed in one of the staves. After several cycles of using (filling and extracting), the bung-hole stave suffers a progressive mechanical damage (transversal cracking, hole deformation, etc.) that affects the barrel hermeticity and therefore increases the volume loss. As the bung-hole hermeticity is often affected, before repairing/remove the complete stave (which is costly and time consuming), a solution is to use a bung made of a wood softer than oak. In this case, the softer bung can then be better “adapted” to the quasi circular shape of the deformed hole. Additionally, it is a very traditional practice in Cuba to adjust the bung into the hole by using a natural innocuous tissue/fiber figure 2(b).
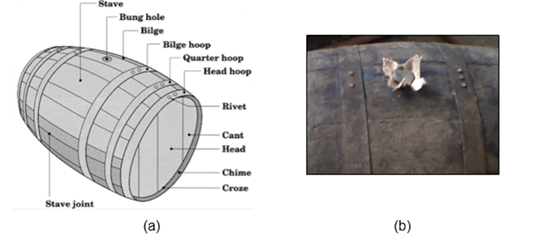
Fig 2 General structural diagram of a wood barrel (a) and softer wood bung adjusted to the hole stave shape by also using a traditional natural tissue to guarantee the barrel hermeticity
Among the Cuban rum producers, it has been extensively discussed about the effect of the bung-hole stave on the volume loss during rum maturation in palletized system. Despite of any study has been yet found/reported about the bung-hole influence, empirical observations have pointed to a somewhat increment of volume loss in palletized system when the barrels keep the bung-hole stave. In palletized system, the barrels without bung-hole stave have instead the bung-hole in the barrel’s head.
In this work, the effect of the barrel bung-hole stave on the volume loss during rum natural ageing in palletized (vertical) system is statistically assessed. Eighty-four traditional 250 L (+/- 10 L) hogshead barrels typically applied in rum natural ageing were studied.
For the 50 % of the barrels the bung-hole stave was removed (without bung-hole: WBH) and the others barrels kept the bung-hole stave (BH). Barrels were jointly stored (randomly assorted) under palletized ageing system during one year into a non-conditioned cellar of the major rum producer in Cuba. The volume loss of the barrels after one year of ageing period was statistically analyzed in order to quantitively evaluate if the bung-hole stave have significant influence on the volume loss increment during palletized ageing system. This study might be interesting not only for rum producers but for alcoholic beverages industry specialists in general.
Materials and methods
Barrels conditioning and sampling
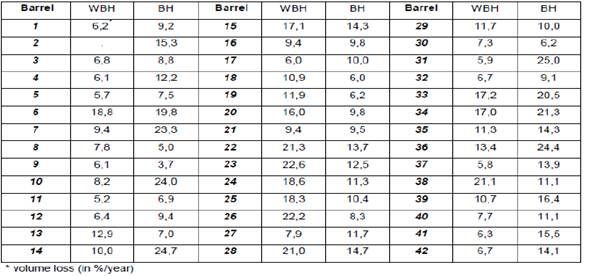
Rum specialists from the major rum producer in Cuba selected eighty-four traditional 250 L (+/- 10 L) hogshead barrels typically applied in rum natural ageing. For the 50 % of the barrels, the bung-hole stave was removed (without bung-hole: WBH) and the others barrels kept the bung -hole stave (BH). Barrels were jointly stored (randomly assorted) under palletized ageing system during one year into a non-conditioned cellar of the major rum producer in Cuba. The volume loss of the barrels was determined by weight-volume difference after one year of ageing period. Table 1 depicts the raw data of volume loss (in %/year) determined for the 84 barrels.
Statistical Analyses
The statistical analyses were developed for the 84 observations presented in table 1 (volume loss of the barrels in %/year) after a year of ageing period. Statistical analyses as well as showed graphs and plots were performed and obtained using CENTURION XV® and ORIGIN® software.
Of particular interest appears the one-way ANOVA test 7 used to evaluate if the volume loss (as an average) differs among barrels with and whitout bug-hole stave in palletized rum ageing system in the cellar. The null hypothesis for this statement is H0: µBH = µWBH with “µi” denoting the average volume loss of barrels with bung-hole stave (BH) and without bung-hole stave (WBH) respectively. Data distribution analysis was performed by applying Normality and t-test methods.8
The ANOVA test and all conclusions derived from it are predicated on three main assumptions:
The residuals follow a normal distribution
The variability (variance) of the residuals is constant (homoscedasticity).
The residuals are independent (randomness).
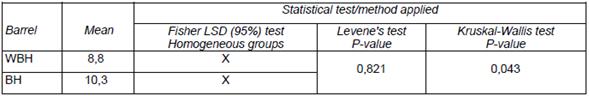
Homoscedasticity (equal variance assumption) was checked using Levene’s test.9 The assumption of independence was also tested in order to evaluate if the volume loss of a given barrel in a group has no information, whatsoever on the volume loss of another barrel in the same or different group. In order to assess if the responses in each group of barrels type (BH and WBH) are coming from different distributions, the Kruskal-Wallis test was used.10 Kruskal-Wallis test verifies the null hypothesis of the volume loss medians of the assessed barrels are the same. Statistical differences between WBH and BH barrels means was performed by applying the multiple rank tests based on the Fisher’s least significant difference (Fisher LSD (95%)).11
Results and discussion
Figure 3 depicts the dispersion plot of the raw data presented in table 1. Based on the experience of rum specialists participating in this study, three regions can be defined from the obtained data. The “Hermeticity failure zone” (figure 3) is considered for barrels which exhibited a volume loss up to 15 %/year (indicated by the arrow). In this case, an obvious leakage occurred during the ageing period thus the volume loss data is not useful as it is affected by external (hermeticity/structural) factors. The “No representative zone” (just involving a few data points) is defined for barrels which presented too low volume loss compared with the volume loss range (6-15 %) for barrels in the specific cellar selected for this study according to the historical records of the rum specialists. Therefore, just the data points located within the “Statistic interest zone” (figure 3) were selected to perform the statistic analyses as represent the average behavior in the studied cellar.
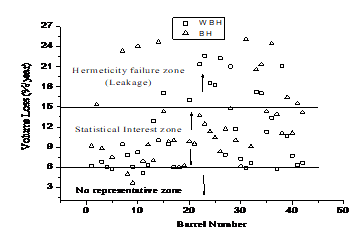
Fig.3 Dispersion plot of the volume loss raw data (table 1) and definition of the statistical interest zone for WBH and BH barrels
Figure 4 presents the plot of ascending sorted raw data of table 1 for BH and WBH barrels.
Fig.4 Ascending sorted arrangement of raw data (Table 1) and definition of the statistical interest zone for WBH and BH barrels
In contrast with figure 3, by applying the ascending data arrangement, it is possible to clearly discriminate de number of data points within each zone and specially those into the statistical interest zone.
From the plotting of figure 4 within the statistical interest zone, a clear trend can be noticed for BH and WBH barrels but following different trajectories. A magnification of the sorted data points of the statistical interest zone for the BH and WBH barrels is presented in figure 5.
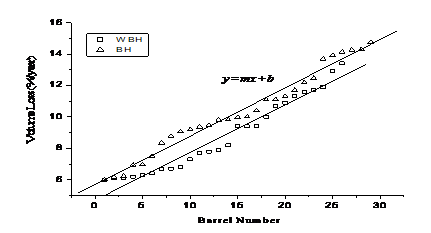
Fig. 5 Magnification and linear fitting of the sorted data points of the statistical interest zone for the BH and WBH barrels
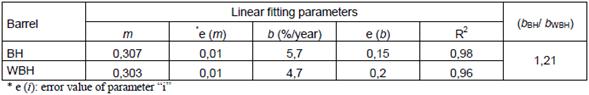
From data plotted in figure 5, it is possible to fit the linear model for each barrel type. Linear fitting parameters are shown in table 2. A satisfactory fitting goodness with regression coefficients over 96% were obtained in both cases. Another interesting aspect to take into account is the slope (m i ) of both linear models which exhibited almost the same value thus indicating that lines are practically parallel. However, found intercepts (b i ) (which in this case denotes the distance between the parallel lines trajectories) present different values.
Prior statistical analysis, differences between the linear models suggests that BH and WBH barrels present different behaviors in terms of volume loss. Based on the fitting results, the linear intercepts ratio (table 2) between barrels points to an increment of volume loss in about 21% when the barrel has the bung-hole stave in vertical position compared with WBH barrels. This result will be statistically confirmed further on.
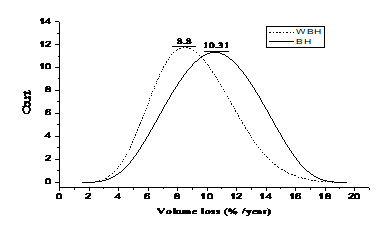
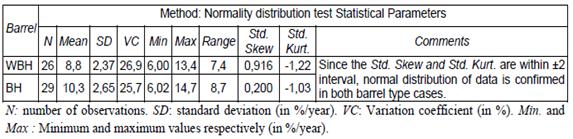
Figure 6 depicts the data distribution of the statistical interest zone data points. Distribution plots were smoothed by applying the fast Fourier Transform method (FFT) on ORIGIN®. The normality distribution of both BH and WBH observation was confirmed (CENTURION XV® ) and the summary of the statistical parameters of the distribution test are depicted in table 3.
Since the standard skew (Std. Skew) and standard Kurtosis (Std. Kurt.) are within ±2 interval, the normal distribution of both BH and WBH data is then confirmed (table 3). The volume loss mean of BH barrels was found in 10,3 %/year which is about 17 % higher than the WBH mean of 8,8 %/year. Statistical differences between the BH and WBH means were confirmed by applying “t” and ANOVA tests which statistical summaries are depicted in tables 4 and 5 respectively.
The P-value was lower than 0,05 thus there is a significant statistical difference between the means of both barrels. The conclusion in both statistical analyses is the same: BH and WBH means are statistically different at 95 % of confidence level. The statistical results (17 % of means difference) confirm the linear fitting intercept ratio between BH and WBH barrels (21 %). In addition, the multiple rank tests based on the Fisher’s least significant difference (Fisher LSD (95%)) test presented in table 6 and plotted in figure 7 also confirms the ANOVA results. The shifted position of the X’s indicates that the of BH and WBH barrels are not homogeneous groups thus significantly different according to Fisher’s LSD (95 %) test.
In short, the Levene’s test assess as null hypothesis of standard deviations of the BH and WBH data points are equal. In this case, the P- value is higher than 0,05 (table 6) thus confirming that there is not statistical difference between the standard deviation of the data from each barrel group at 95 % of confidence level.
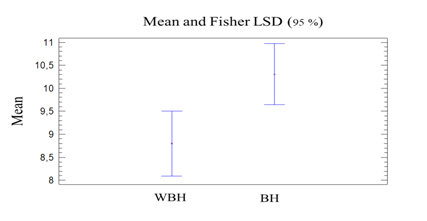
Fig.7 Plot of the multiple rank test based on Fisher LSD (95%) method for BH and WBH barrels comparison
The Kruskal-Wallis test (table 6) is applied in this case to evaluate the null hypothesis that the medians coming from the of the BH and WBH data points are equal. Since the P-value is lower than 0,05, the statistical differences between the medians of BH and WBH barrels is also confirmed at 95% of confidence level.
Based on the statistics results, the bung-hole stave produces and increment of the volume loss in about 17% compared with barrels without bung-hole staves when palletized rum ageing system is applied. However, beyond statistical facts, the phenomenological explanation of this behavior needs to be further studied and experimentally demonstrated. Actually, mass transfer phenomena in barrels during alcoholic beverages ageing process still being a current and challenging research topic.1
Figure 8 depicts a general diagram of a transversal-cut view of a bung-hole stave in a barrel stored in vertical position as palletized ageing system. The soft-wood bung is inserted in the hole and adjusted with a natural tissue (dash line).
The equivalent mass diffusion coefficient of the small bung region in the barrel (
) (hole deformation, soft wood bung and adjusting tissue) is much higher than the equivalent mass diffusion coefficient for an oak barrel without bung-hole stave (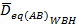
) vertically stored.
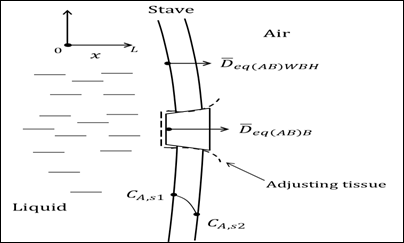
Fig.8 General diagram of a transversal-cut view of a bung-hole stave in a barrel section stored in vertical position (palletized ageing system)
The evaporation of the liquid into the barrel to the surrounding air, mainly (but not exclusively) occurs due to diffusional transport mechanisms trough the wood of the staves and joints which can be modeled by the Fick’s law for one-dimensional diffusion of a specie “A” (liquid) through a planar medium “B” (wood) at steady state which mathematically can be described by eq. (1)
With
N A,X : Molar rate in “x” direction (in mol/s)
D AB : Mass diffusion coefficient (in m2/s)
: Surface area (in m2)
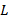: Directional mass transport dimension (in m)
C A,s1 and C A,s2: Concentration of specie “A” on surfaces S1 and S2 respectively (in mol/m3)
However, the barrel is not a solid/continuous wooden truncated prolate spheroid but an assembled structure composed by several wood pieces which are mechanically jointed by metallic hoops (figure 2). Based on this, since the material homogeneity and continuous medium in the barrel is not fulfilled, the eq.1 can be modified as follows:
Where
: Equivalent mass diffusion coefficient of the barrel (in m2/s)
In this case, represents the combined contribution of mass diffusion coefficients of liquid evaporation considering not only the diffusion through the barrel wood but also the liquid evaporation by capillary conduction of the barrel joints between staves, cants, croze and the bung-hole with the adjusting tissue/fiber. (figure 2 (a) and (b)). Additionally, as above statistically demonstrated, the volume loss ratio between BH and WBH barrels can be written as
V LOSS(BH) : Mean of volume loss in barrels with bung-hole stave (in %/year)
V LOSS(WBH) : Mean of volume loss in barrels without bung-hole stave (in %/year)
As the volume loss is a direct function of N A,X , and assuming the same concentration gradient in the barrel for the liquid-wood-air equilibrium system stablished, equations (2) and (3) can be then combined as
: Equivalent mass diffusion coefficient of the BH barrel (in m2/s)
: Equivalent mass diffusion coefficient of the WBH barrel (in m2/s)
L BH and L WBH : Stave thickness of BH and WBH barrels respectively (in m)
S BH and S WBH : Surface area of BH and WBH barrels respectively (in m2)
Actually, the only difference between the studied BH and WBH barrels is the bung-hole stave. The barrel bung is a truncated cone of about dB=5 cm of average diameter and thickness of L B = 2 cm. Therefore, the surface area of the barrel bung can be roughly assumed as S B = 0,002 m2.
On the other hand, a 250L traditional hogshead traditional barrel in vertical position (one of the barrel’s head is not in contact with the liquid) have about 1,96 m2 of surface area and its staves’ thickness in the range of 2-2,5 cm. 6
Considering equal thickness of the bung and staves (L B = L BH = L WBH ) and transforming eq. (5) by disaggregating the effect of the bung diffusional process (figure.8) gives:
: Equivalent mass diffusion coefficient of the bung region (in m2/s)
S B : Surface area of the barrel bung (in m2)
As S B (( S WBH , then it is possible to assume that (S WBH - S B ) ( S WBH
Thus reordering eq. (6) gives
Then
From eq. (7) and the statistical results at the studied conditions, it was found that the equivalent mass diffusion coefficient of the small bung-hole region in the barrel () is about 167 times higher than the equivalent mass diffusion coefficient of an entire oak barrel without bung-hole stave vertically standing during ageing process in palletized system. The combined result of the stave hole deformation, the softer wood used for making the bung and the capillary effect of the adjusting tissue generates a significant increment in equivalent mass diffusion coefficient around the bung-hole region in the BH barrels. However, theoretical arguments must be further studied and experimentally confirmed/corrected.
Conclusions
In this work, the effect of the barrel bung-hole stave on the volume loss during rum natural ageing in palletized (vertical) system was statistically determined. The volume loss of 84 traditional 250 L hogshead barrels (50% with and 50% without bung-hole stave) after a year of ageing period in a non-conditioned cellar was analized. The statistical interest data was classified and normal distribution of the data for the two studied barrels groups was confirmed by applying Normality-test. Additionally, the homoscedasticity of both barrels’ groups data was confirmed by the Levene’s test.
Found results by performing One-way ANOVA, Fisher’s least significant difference (Fisher LSD (95%)) and Kruskal-Wallis statistical tests confirmed that the bung-hole stave significantly increases the volume loss during rum natural ageing in palletized system in about 17% compared to barrels without bung-hole stave at the studied conditions with a confidence level of 95%.
Based on theoretical estimations, it was found that the equivalent mass diffusion coefficient of the small bung-hole region in the barrel is about 167 times higher than the equivalent mass diffusion coefficient of an oak barrel without bung-hole stave vertically standing during ageing process in palletized system. The combined result of the stave hole deformation, the softer wood used for making the bung and the capillary effect of the adjusting tissue generates a significant increment in equivalent mass diffusion coefficient around the bung-hole region.
