INTRODUCTION
With the great development of RIB facilities, regions away from stability in the nuclear chart of the elements become accessible to experimentation, leading to a complete map of the limits of bound matter in some areas [1]. This is the case on the proton rich side, where the proton drip line was almost completely defined up to Z=83. In this process, proton radioactivity played an important role, especially for Z> 50 where one proton emitters from ground and isomeric states were produced, in fusion evaporation reactions of neutron deficient nuclei [2]. The cross sections for these process are very low, and the half-lives very short, leading only to a narrow energy window, that can be accessible with our present detection apparatus.
For charges below 50, the extremes of stability are being accessed by multifragmentation reactions of exotic beams, and also proton radioactive nuclei are being found [3]. The emission of two protons has been also observed in this intermediate mass region with the first case of 45Fe, and afterwards for other nuclei [4].
Nuclei at the limits of stability are fundamental to understand the nuclei synthesis of the elements. An overview of the main astrophysical processes shows that the vast majority of reactions, involve quite unstable nuclei. The path for the rapid proton capture processes might involve nuclei close to the proton drip-line [5], and in most cases, a stable p-nuclide will be synthesized through a quite unstable progenitor formed at the end stage of the evolution of massive stars, where there are enough seed nuclei, and high temperatures, for the capture reactions to take place.
Thus, the location of the proton drip line constrains the path of the process of the nucleosynthesis in explosive astrophysical scenarios such as in supernovae and X-ray bursters [6], this path, however, depends on the level structure and existence of resonances in proton rich nuclei. Many reaction chains along the rp processes can go via direct or resonance capture of the proton, therefore, the knowledge of the properties of the excited states is also required.
Nuclear astrophysics models strongly depend on a very precise determination of the reaction rates and reaction energies [7]. For the calculation of the reaction rates some of the nuclear ground state properties, like masses, and deformations, need to be known. Moreover, the evaluation of the reverse (ɣ, p) reaction with respect to proton emission, is of key importance in the proton networks.
Since drip line nuclei are very unstable and short-lived, direct experiments are still a challenge, and the cross sections for most reactions are unknown. The energies can be obtained in some cases from nuclear masses, but particle-decay spectroscopy is for most of these nuclei the only way to determine their properties, like the separation energy and the identification of the decaying states. The observation of proton emission from nuclei lying beyond the proton drip line, and its theoretical interpretation has made possible to access the nuclear structure properties in the neutron deficient region of the nuclear chart [8,9]. It has also provided an indirect way to determine separation energies and identifying some cases the excitation spectrum of the emitter.
The previous discussion points out the strong link between astrophysical properties needed for explosive scenarios, and the properties of exotic proton rich nuclei, short lived and only known from their decay data.
It is the purpose of this work to discuss recent developments in the study of proton rich nuclei, and their relevance to nuclear astrophysics. From the interpretation of their decay data by proton emission, we will identify properties of their spectra and shape, and deduce constraints to the astrophysical processes.
The spectra and shape of proton rich nuclei
In the experimental observation of proton decay, the energy of the emitted proton, half lives and branching ratios for decay to excited states are measured, except in the case of multifragmentation production of these exotic nuclei, where only values for the half-lives can be obtained.
A model that can reproduce these experimental observables, can also assign the spins and parities of the decaying states, and the nuclear shape parameters.
Consequently, these decay modes can be used to probe the wave functions of the nuclear levels involved and to study also nuclear shell effects. The non-adiabatic -quasi-particle model [10], has been very successful in this context, and was able to explain many aspects of the structure of odd-even nuclei, with an odd number of protons [11], as well as odd-odd nuclei [12], including the breaking of axial symmetry [13]. The model, incorporates the excitation spectrum of the daughter nucleus and the residual interaction in a consistent way, and we will discuss below a few cases which might be relevant in the nuclear astrophysics context.
An interesting example that shows the capability of the model to determine the spectra and shape parameters, is given by the study of 151Lu.In a recent experiment [9], using recoil proton tagging, the lifetime of the first excited state above the proton emitting ground state of 151Lu, as well as higher-lying longer-lived states have been measured using ɣ ray coincidence techniques. Theoretical calculations based on the non-adiabatic quasi-particle model, coupling the quasi-particles with the spectrum of the daughter nucleus, suggest [9] that the proton emitting ground state of 151Lu is a h11/2-, with a mildly oblate quadrupole deformation of β ~ - 0.11. The life time of the first excited state, which decays by an electromagnetic ɣ ray transition was also calculated with the same description of the nucleus, showing the consistence of the model and giving the best evidence to date for proton emission from an oblate nucleus. For details see reference [9].
This example, is an interesting determination of shape parameters, and spectrum of drip-line nuclei, through the observation of proton decay.
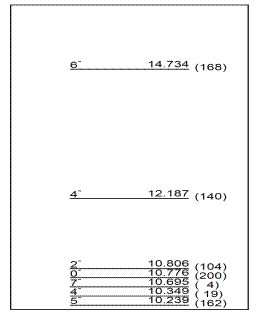
Figure 1 Calculated excited states in 18Ne with a total width smaller than 200 keV and a branching ratio for decay to unbound states larger than 0.7. The spin and parity, and energy in MeV of the decaying levels are given in the figure. The width of the resonances in KeV, are shown in parenthesis.
An important nucleus for astrophysical calculation is 18Ne, since the breakout path from the hot CNO cycle involve sequences of alpha particle reactions that proceed through the 18Ne resonances, above the alpha decay threshold. Also, the reaction 17F(p,ɣ)18Ne is particularly important for the rp-process path, and it is just the inverse of proton decay from a resonance in18Ne. The determination of resonances in 18Ne is then an important issue to obtain the reaction rates, but they are not easy to observe experimentally [14].
The nucleus18Ne exhibits two proton radioactivity [15,16], and beside the simultaneous two proton emission from a 1- state at 6.15 MeV, there is also at higher excitation energy a strong branch ~30%, for sequential emission of one proton after the other, going through excited states of 17F [17] before reaching the final daughter nucleus 16O in the ground state.
This is quite surprising, since simple barrier penetration considerations would favour a decay with the largest amount of energy being carried out by the first proton, thus to the ground state of 17F that is bound, and consequently the second proton would not be emitted. A smaller percentage decays directly by simultaneous two proton emission. A theoretical analysis in terms of sequential decay, can lead to the identification of possible excited states, candidates for the emission of the second proton.
Since 18Ne is a spherical nucleus, the half-life can be calculated according to scattering theory, from the knowledge of the proton state and the spectroscopic factor. The latter, can be determined from a standard shell model calculation with a realistic interaction. We have used the interaction of reference [18] which was fitted to the experimental excitation energies, and reproduces the experimental data for all nuclei. The calculation shows that there are excited states of negative parity at quite high energies in 18Ne, which are very narrow, and prefer to decay by one proton emission to the excited states than to the ground state of the daughter 17F, thus becoming possible candidates for the emission of a second proton in a sequential two-proton decay process. The calculation of branching ratios for decay to unbound proton states in 17F, and selecting the states that have a narrow width and a large branching ratio predicts the existence of negative parity states at quite high energy in 18Ne, and are presented in figure 1. Some of these states were confirmed in the experiment of Ref. [15]. This study shows the power of proton emission to identify the spectra of proton radioactive nuclei.
In the region of production of medium heavy isotopes, N=Z nuclei play an important role, since rp processes seem to follow the N=Z line up to the neighbourhood of the double shell closure N=Z=50 nucleus 100Sn. The time scale for the reactions is controlled by the existence of waiting points isotopes,64Ge, 68Se, 72Kr, and the knowledge of the proton separation energies, and half-lives of the neighbour nuclei, would allow to establish the most probable path through these nuclei. In a recent multifragmentation experiment at Riken [19], Rb isotopes, 72Rb, and 73Rb were produced, which are in the vicinity of 72Kr. Both nuclei are proton emitters, being the half-life for 72Rb measured, but 73Rb was not observed, so only an upper limit for its half life was predicted. They are expected to have a large quadrupole deformation [20].
Decay of 73Rb was calculated in a similar way as in the previous example of 151Lu, since it is the decay of an odd-even nucleus. From experiment only an upper bound of 80 ns is known for the half-life, and to fulfil this constraint we found from our calculation, that the escaping energy of the proton could only be above 600 keV, in very good agreement with the recent evaluation of atomic masses [21,22], Since the separation energy, is the symmetric of the escaping energy, we have obtained in this way a lower bound for the proton separation energy Sp in 73Rb.
This has important implications for nuclear astrophysics, since the half-life of a waiting point depends exponentially on the Sp of the intermediate nucleus. It is possible to show in a x-ray burst model [23] that this limit for Sp prevents the72Kr waiting point to be bypassed by a two proton capture in the rp path, therefore staying as a strong waiting point.
The isotope 72Rb is an interesting example of proton emission from a deformed odd-odd nucleus. The emission of one proton in such nuclei, is quite dependent on the state of the unpaired neutron. The daughter nucleus will have an odd number of nucleons, and its angular momentum is determined by the Nilsson level occupied by the odd neutron. Different values of this angular momentum, will allow different values of the angular momentum of the escaping proton [24].
The theoretical interpretation of proton emission from odd-odd based on the adiabatic quasi-particle model, is also well established [12], and includes the proper treatment of residual pairing and neutron-proton interactions. It is possible to single out the angular momentum and parity of the ground state of these emitters and estimate the effect of the residual np interaction.
The half-life of 72Rb was measured [19] and found to be 103( 22) ns. The theoretical interpretation of this observable implied for the energy of the proton a value of order of 80eV also in close agreement with the atomic masses evaluation of the separation energy [21,22],
CONCLUSIONS
In conclusion, the theoretical interpretation of the experimental data on proton decay from proton rich nuclei, provides the possibility to predict the properties of the decaying state, the nuclear deformation, the level structure and a possible existence of isomeric states. It also provides a way to impose directly from the experiment, constraints on the separation energy.
This information, is quite relevant for nuclear astrophysics studies, in particular for the region of charges Z<50, were the rp process involves proton drip-line nuclei, that cannot be probed directly from experiment. Special attention is given to decay processes that involve nuclei in the vicinity of a waiting point, since it is the knowledge of the proton separation energies that can decide the flow through these nuclei.